



Next: General idea of the
Up: Determination of the trigger
Previous: Role of T0
Contents
Kopytine's homepage
Distribution of the number of tracks that hit the spectrometer's
acceptance deviates
from the Poissonian one due to the variation of the Poissonian mean event to
event, and due to two-particle and multiparticle correlations.
Realizing that, it is nevertheless useful to recall that in the Poissonian
case with average 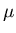 ,
,
 .
Upon comparison with the ratio of double to single track events we
see in the central trigger runs of our experiment, it is clear that
even in Pb+Pb, the spectrometer (even in the low angle setting, due to
the ``jaws'')
presents a target which is difficult to hit
(
.
Upon comparison with the ratio of double to single track events we
see in the central trigger runs of our experiment, it is clear that
even in Pb+Pb, the spectrometer (even in the low angle setting, due to
the ``jaws'')
presents a target which is difficult to hit
(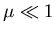 ).
I use
).
I use
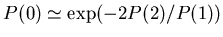 |
(20) |
as a measure of the probability that the spectrometer
has zero tracks.
(In case of the Poissonian law, the equation (4.18)
would be exact.
The current discussion however does not pursue more than qualitative
understanding.)
Table 4.2.3 summarizes the Poissonian estimates of 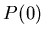 .
.
Table:
The estimated
fraction of events that do not create tracks in the spectrometer.
It has been obtained
according to the Poissonian law,
 ,
based on the DST information.
,
based on the DST information.
| momentum |
+ 4 GeV |
+ 8 GeV |
- 4 GeV |
- 8 GeV |
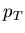 setting setting |
low |
high |
low |
high |
low |
high |
low |
high |
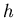 |
0.76 |
0.91 |
0.71 |
0.95 |
0.77 |
0.92 |
0.72 |
0.96 |
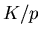 |
0.96 |
0.97 |
0.93 |
0.97 |
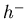 |
0.99 |
0.97 |
0.99 |
|
Had it been easy to satisfy (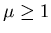 ),
the MUL1 requirement would not have been
considered important component of the centrality trigger.
Therefore, MUL1 needs to be taken into account
for determination of the trigger centralities.
),
the MUL1 requirement would not have been
considered important component of the centrality trigger.
Therefore, MUL1 needs to be taken into account
for determination of the trigger centralities.




Next: General idea of the
Up: Determination of the trigger
Previous: Role of T0
Contents
Mikhail Kopytine
2001-08-09
![]() ),
the MUL1 requirement would not have been
considered important component of the centrality trigger.
Therefore, MUL1 needs to be taken into account
for determination of the trigger centralities.
),
the MUL1 requirement would not have been
considered important component of the centrality trigger.
Therefore, MUL1 needs to be taken into account
for determination of the trigger centralities.